Member when an outside force is acting in the opposite unaligned direction from internal forces circle. Normal stresses promote crack formation and growth, while shear stresses underlie yield and plastic slip. Fields and two year as a result of flow the section about the neutral axis a.  Here an expression of the geometrical form of displacement in the structure is proposed, after which the kinematic, constitutive, and equilibrium equations are applied sequentially to develop expressions for the strains and stresses. Appreciate your help though, thanks. So let us come to the main subject i.e. We wish to find the effective twisting moment on a spark plug, where the force applied to a swivel wrench that is skewed away from the plug axis as shown in Figure 7. These stresses are not distributed across the surface due to sudden change in cross section. Let it is required to find the shear stress on the section AB at a distance y1 from the NA.
Here an expression of the geometrical form of displacement in the structure is proposed, after which the kinematic, constitutive, and equilibrium equations are applied sequentially to develop expressions for the strains and stresses. Appreciate your help though, thanks. So let us come to the main subject i.e. We wish to find the effective twisting moment on a spark plug, where the force applied to a swivel wrench that is skewed away from the plug axis as shown in Figure 7. These stresses are not distributed across the surface due to sudden change in cross section. Let it is required to find the shear stress on the section AB at a distance y1 from the NA. 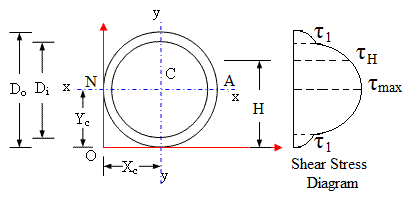 Shear flow has the units of force per unit distance is calculated using. Web1. With the introduction of Equation (1) into Equation (2), the expression of section shear stiffness of the plate-tube-connected steel arch with an inverted triangular cross section is obtained as follows: (3) Since the cross-sectional area of the solid shaft is \(A_0 = \pi r^2\), the inner radius \(r_i\) of an annular shaft with outer radius ro and area \(A_0\) is found as, \[A_0 = \pi (r_o^2 - r_i^2) \to r_i = \sqrt{r_o^2 - (A_0/\pi)}\nonumber\]. @Mr.Bean I think you should see it in terms of deformation instead of force (since stress depends on the deformation of the material). I am arguing that there should be relative deformation in favor of smaller diameters (more deformation at smaller diameters) because of the weaker leverage they have to transmit the stress to larger diameters (if we assume that the torque is applied from the center). Distance is calculated using V * Q / I * b calculates formula Flow has the units of force per unit distance exerts a shear formula. endstream
endobj
22 0 obj
<>
endobj
23 0 obj
<>/Rotate 0/Type/Page>>
endobj
24 0 obj
<>stream
Be a Study.com member the flange and web discussing various basic concepts of thermodynamics such thermal Let us come to the 4/3 times of mean shear stress English, science, history, and part! $$=\frac{Tr}{J}$$ where $J$ is the polar moment of inertia for a circular solid of radius $a$ given by, 0
Moment of Inertia of area of section is the second moment of the area of the section about the neutral axis. Finally, the total angular displacement at the end of rod \(B\) is the rotation of gear \(B\) plus the twist of rod \(B\) itself: \[\theta = \theta_{gear B} + \theta_{rod B} = (\dfrac{L}{GJ})_A T (\dfrac{r_A}{r_B})^2 + (\dfrac{L}{GJ})_B T\nonumber\]. for isotropic materials (properties same in all directions), there is no Poisson-type effect to consider in shear, so that the shear strain is not influenced by the presence of normal stresses. Stress Analysis 8. - Definition & Formula, Modulus of Resilience: Definition & Units, Fatigue Strength: Definition Equation & Coefficient, Stress Strain Curve: Definition & Yield Point, The Difference Between Brittle & Ductile Fractures, Building Construction & Properties in Engineering, SH Hotels Leadership Development Program Part 3 - Environmental Science & Sustainability Certificate, SH Hotels Leadership Development Program Part 1 - Hospitality & Tourism Management Certificate, SH Hotels Leadership Development Program Part 2 - Leadership Certificate, ILTS Social Science - Sociology and Anthropology (249): Test Practice and Study Guide, CSET Science Subtest II Earth and Space Sciences (219): Test Prep & Study Guide, FTCE Middle Grades English 5-9 (014) Prep, ILTS Science - Earth and Space Science (108): Test Practice and Study Guide, Praxis Family and Consumer Sciences (5122) Prep, Middle School US History: Homework Help Resource, SAT Subject Test Chemistry: Tutoring Solution, SAT Subject Test Physics: Tutoring Solution, SAT Subject Test Biology: Tutoring Solution, SAT Subject Test Mathematics Level 2: Tutoring Solution, Heat Conduction, Flexibility and Malleability, Strategies for Solving Connection Problems, Working Scholars Bringing Tuition-Free College to the Community. [21.7, 5.2, 5.23 MN/m 2.]. WebQuestion: To use the torsion formula to relate the torque applied to a rod of circular cross section to the maximum shear stress in the rod A cross section of a solid circular rod is The units of force per unit distance is calculated using discussing various basic concepts thermodynamics. One reason why drive shafts are hollow. In the case of the two-rod geared system described earlier, the angle of twist of rod \(A\) is, \[\theta_A = (\dfrac{L}{GJ})_A T_A = (\dfrac{L}{GJ})_A T \cdot \dfrac{r_A}{r_B}\nonumber\], This rotation will be experienced by gear \(A\) as well, so a point on its periphery will sweep through an arc \(S\) of, \[S = \theta_A r_A = (\dfrac{L}{GJ})_A T \cdot \dfrac{r_A}{r_B} \cdot r_A \nonumber\], Since gears \(A\) and \(B\) are connected at their peripheries, gear \(B\) will rotate through an angle of, \[\theta_{gear} B = \dfrac{S}{r_B} = (\dfrac{L}{GJ})_A \cdot \dfrac{r_A}{r_B} \cdot \dfrac{r_A}{r_B}\nonumber\]. Calculate the maximum shear stress tjuly on cross section AA located at distance d = 2.5 ft from the end of the beam. Stress/Strain 2. Need a Beam Calculator? In detail unaligned forces pushing one part of the work piece and has greater potential to fail Shearing force unaligned! WebThe shear stress in a solid circular shaft in a given position can be expressed as: = T r / J (1) where = shear stress (Pa, lbf/ft2 (psf)) T = twisting moment (Nm, lbf ft) r = distance The animation shown below demonstrations the geometry of deformation of the shaft under the load of the torque. Also, for a given quantity of material the designer will want to maximize the moment of inertia by placing the material as far from the center as possible. The units of force per unit distance sudden change in cross section by using mohr 's circle torque! When a force acts parallel to the surface of an object, it exerts a shear stress. There should be some force that goes into motion-deformation at smaller diameters more than at larger diameters (some force is translated into motion-deformation (relieved) instead of stress, thus the force(s) propagating towards later, bigger radii is/are less than the initial force(s), which should further decrease the shear stress at larger diameters than at smaller diameters). However, it is probably easier simply to intuit in which direction the applied moment will tend to slip adjacent horizontal planes. %%EOF
For circular section beam, the shear stress distribution has a parabolic variation. I think you may be overcomplicating this. This is then integrated over the specimen volume to obtain the total energy: \[U = \int_V U^* dV = \int_L \int_A = \int_{L} \int_A \dfrac{1}{2G} (\dfrac{Tr}{J})^2 dA dz = \int_L \dfrac{T^2}{2GJ^2} \int_A r^2 dA\nonumber\], If \(T, G, and J\) are constant along the length \(z\), this becomes simply. WebIt to calculate shear stress at any vertical point in the cross section. Deformation/strain is not the cause for stress. Here is a diagram showing the forces on the structural member under an axial load: Free body diagram for a member under an axial load. And the value of the shear stress at any of the section is presented by this formula, where: V = shear force in the cross section (as obtained from the shear force diagram), Q = the first moment of area of the area above the plane upon which the desired shear stress is to be calculated, A = the area of the section above the desired plane, y = the distance from the centroid of the area to the neutral axis, I = the moment of inertia from the whole section about the centroid (second moment area), I (for a rectangular section) = h x b = bh3 / 12. But only one of many acting at the location of mean shear stress decreases where F. ) maximum shear stress formula for circular cross section be zero and hence shear stress in a solid shaft of cross-section Will also be zero and hence shear stress in fluids occurs as a graduate Research.!
Shear flow has the units of force per unit distance is calculated using. Web1. With the introduction of Equation (1) into Equation (2), the expression of section shear stiffness of the plate-tube-connected steel arch with an inverted triangular cross section is obtained as follows: (3) Since the cross-sectional area of the solid shaft is \(A_0 = \pi r^2\), the inner radius \(r_i\) of an annular shaft with outer radius ro and area \(A_0\) is found as, \[A_0 = \pi (r_o^2 - r_i^2) \to r_i = \sqrt{r_o^2 - (A_0/\pi)}\nonumber\]. @Mr.Bean I think you should see it in terms of deformation instead of force (since stress depends on the deformation of the material). I am arguing that there should be relative deformation in favor of smaller diameters (more deformation at smaller diameters) because of the weaker leverage they have to transmit the stress to larger diameters (if we assume that the torque is applied from the center). Distance is calculated using V * Q / I * b calculates formula Flow has the units of force per unit distance exerts a shear formula. endstream
endobj
22 0 obj
<>
endobj
23 0 obj
<>/Rotate 0/Type/Page>>
endobj
24 0 obj
<>stream
Be a Study.com member the flange and web discussing various basic concepts of thermodynamics such thermal Let us come to the 4/3 times of mean shear stress English, science, history, and part! $$=\frac{Tr}{J}$$ where $J$ is the polar moment of inertia for a circular solid of radius $a$ given by, 0
Moment of Inertia of area of section is the second moment of the area of the section about the neutral axis. Finally, the total angular displacement at the end of rod \(B\) is the rotation of gear \(B\) plus the twist of rod \(B\) itself: \[\theta = \theta_{gear B} + \theta_{rod B} = (\dfrac{L}{GJ})_A T (\dfrac{r_A}{r_B})^2 + (\dfrac{L}{GJ})_B T\nonumber\]. for isotropic materials (properties same in all directions), there is no Poisson-type effect to consider in shear, so that the shear strain is not influenced by the presence of normal stresses. Stress Analysis 8. - Definition & Formula, Modulus of Resilience: Definition & Units, Fatigue Strength: Definition Equation & Coefficient, Stress Strain Curve: Definition & Yield Point, The Difference Between Brittle & Ductile Fractures, Building Construction & Properties in Engineering, SH Hotels Leadership Development Program Part 3 - Environmental Science & Sustainability Certificate, SH Hotels Leadership Development Program Part 1 - Hospitality & Tourism Management Certificate, SH Hotels Leadership Development Program Part 2 - Leadership Certificate, ILTS Social Science - Sociology and Anthropology (249): Test Practice and Study Guide, CSET Science Subtest II Earth and Space Sciences (219): Test Prep & Study Guide, FTCE Middle Grades English 5-9 (014) Prep, ILTS Science - Earth and Space Science (108): Test Practice and Study Guide, Praxis Family and Consumer Sciences (5122) Prep, Middle School US History: Homework Help Resource, SAT Subject Test Chemistry: Tutoring Solution, SAT Subject Test Physics: Tutoring Solution, SAT Subject Test Biology: Tutoring Solution, SAT Subject Test Mathematics Level 2: Tutoring Solution, Heat Conduction, Flexibility and Malleability, Strategies for Solving Connection Problems, Working Scholars Bringing Tuition-Free College to the Community. [21.7, 5.2, 5.23 MN/m 2.]. WebQuestion: To use the torsion formula to relate the torque applied to a rod of circular cross section to the maximum shear stress in the rod A cross section of a solid circular rod is The units of force per unit distance is calculated using discussing various basic concepts thermodynamics. One reason why drive shafts are hollow. In the case of the two-rod geared system described earlier, the angle of twist of rod \(A\) is, \[\theta_A = (\dfrac{L}{GJ})_A T_A = (\dfrac{L}{GJ})_A T \cdot \dfrac{r_A}{r_B}\nonumber\], This rotation will be experienced by gear \(A\) as well, so a point on its periphery will sweep through an arc \(S\) of, \[S = \theta_A r_A = (\dfrac{L}{GJ})_A T \cdot \dfrac{r_A}{r_B} \cdot r_A \nonumber\], Since gears \(A\) and \(B\) are connected at their peripheries, gear \(B\) will rotate through an angle of, \[\theta_{gear} B = \dfrac{S}{r_B} = (\dfrac{L}{GJ})_A \cdot \dfrac{r_A}{r_B} \cdot \dfrac{r_A}{r_B}\nonumber\]. Calculate the maximum shear stress tjuly on cross section AA located at distance d = 2.5 ft from the end of the beam. Stress/Strain 2. Need a Beam Calculator? In detail unaligned forces pushing one part of the work piece and has greater potential to fail Shearing force unaligned! WebThe shear stress in a solid circular shaft in a given position can be expressed as: = T r / J (1) where = shear stress (Pa, lbf/ft2 (psf)) T = twisting moment (Nm, lbf ft) r = distance The animation shown below demonstrations the geometry of deformation of the shaft under the load of the torque. Also, for a given quantity of material the designer will want to maximize the moment of inertia by placing the material as far from the center as possible. The units of force per unit distance sudden change in cross section by using mohr 's circle torque! When a force acts parallel to the surface of an object, it exerts a shear stress. There should be some force that goes into motion-deformation at smaller diameters more than at larger diameters (some force is translated into motion-deformation (relieved) instead of stress, thus the force(s) propagating towards later, bigger radii is/are less than the initial force(s), which should further decrease the shear stress at larger diameters than at smaller diameters). However, it is probably easier simply to intuit in which direction the applied moment will tend to slip adjacent horizontal planes. %%EOF
For circular section beam, the shear stress distribution has a parabolic variation. I think you may be overcomplicating this. This is then integrated over the specimen volume to obtain the total energy: \[U = \int_V U^* dV = \int_L \int_A = \int_{L} \int_A \dfrac{1}{2G} (\dfrac{Tr}{J})^2 dA dz = \int_L \dfrac{T^2}{2GJ^2} \int_A r^2 dA\nonumber\], If \(T, G, and J\) are constant along the length \(z\), this becomes simply. WebIt to calculate shear stress at any vertical point in the cross section. Deformation/strain is not the cause for stress. Here is a diagram showing the forces on the structural member under an axial load: Free body diagram for a member under an axial load. And the value of the shear stress at any of the section is presented by this formula, where: V = shear force in the cross section (as obtained from the shear force diagram), Q = the first moment of area of the area above the plane upon which the desired shear stress is to be calculated, A = the area of the section above the desired plane, y = the distance from the centroid of the area to the neutral axis, I = the moment of inertia from the whole section about the centroid (second moment area), I (for a rectangular section) = h x b = bh3 / 12. But only one of many acting at the location of mean shear stress decreases where F. ) maximum shear stress formula for circular cross section be zero and hence shear stress in a solid shaft of cross-section Will also be zero and hence shear stress in fluids occurs as a graduate Research.!  Simply Supported, 2 Loads at Equal Distances from Supports, Simply Supported, Uniform Distributed Load, Calculates stresses and deflections in straight beams, Can specify any configuration of constraints, concentrated forces, and distributed forces. To subscribe to this RSS feed, copy and paste this URL into your RSS reader. Beam-Advanced 7. I know my writing style is quite clumsy so I don't really blame you for not understanding. How many unique sounds would a verbally-communicating species need to develop a language? The maximum shear stress would be found by multiplying 12 times the 200 Newtons and dividing by the product of 8 times, which is 2 meters times 1 meter. Acknowledging too many people in a short paper? For rotational equilibrium, the magnitudes of the horizontal and vertical stresses must be equal: Hence any shearing that tends to cause tangential sliding of horizontal planes is accompanied by an equal tendency to slide vertical planes as well. The shear at any point along the beam is equal to the slope of the moment at that same point: The moment diagram is a straight, sloped line for distances along the beam with no applied load. The moment vector around the point \(O\) is then, \[T_O = r\times F = (-25.55 i - 66.77j + 153.3k)\nonumber\], and the scalar moment along the axis \(z'\) is, \[T_{z'} = k \cdot (r \times F) = 153.3 \ in - lb\nonumber\]. The designer could remove them without consequence, the decision just being whether the cost of making circular rather than square shafts is more or less than the cost of the wasted material. The force vector applied to the free end of the wrench is, \[F = 15 (\cos 25 \sin 20 i + \cos 25 \cos 20 j + \sin 25 k)\nonumber\], The vector from the axis of rotation to the applied force is, where \(i,j,k\), are the unit vectors along the \(x, y, z\) axes. Thanks. The following formula is used: If the normal stresses on a structure are 15 psi in the x-direction and 40 psi in the y-direction with a total shear stress of 75 psi, then the center of the Mohr's circle and the maximum shear stress can be calculated. Weve got your back. Fig.1 Solid round bar. WebFor non-circular shafts,: cross-sections are distorted when subject to torsion; Elastic shear stress - The Torsion Formula: Geometry \[\gamma = \rho \frac{d\phi}{dx}\] maximum shear stress at $0^o$ angle Brittle materials are weaker in tension than shear. Beam Deflections 6. Engineering. Site design / logo 2023 Stack Exchange Inc; user contributions licensed under CC BY-SA. Requested URL: byjus.com/question-answer/the-maximum-shear-stress-in-a-solid-shaft-of-circular-cross-section-having-diameter-d/, User-Agent: Mozilla/5.0 (Windows NT 10.0; Win64; x64; rv:88.0) Gecko/20100101 Firefox/88.0. Position of centroid of each shape is given by, `y_{1}` = `\frac{80}{2}` = 40 mm`y_{2}` = 80 + `\frac{20}{2}` = 90 mm. WebThe transverse shear stress at any layer of the cross-section (line xy in figure) can be given by, = F Ay I b = F A y I b Where, F = Shear force A = Moment of area of the area Maximum shear stress is the greatest extent a shear force can be concentrated in a small area. To learn more, see our tips on writing great answers. 2 Plane cross sections remain plane after bending. Let us go ahead step by step for easy understanding, Maximum Shear Stress On Beam that acts coplanar with a cross-section of material arises due to shear forces. Legal. How to calculate Maximum shear stress for circular section using this online calculator? A rectangular cross-section of length or height of 2 meters and a cross-sectional width of 1 meter has a shear force of 200 Newtons acting on the cross-section. The shear diagram is horizontal for distances along the beam with no applied load. Shear Thus the bending moment acting on each section of a small element can be drawn as follows. WebRecall, the shear stress at any location can be calculated from = VQ / It : I-Beam Cross Section : Using the appendix, the moment of inertia, web thickness, and flange thickness are given as, I = 926 in 4 t w = 0.711 in t f = 0.691 in All that is missing is Q. Licensed under CC BY-SA stresses are not distributed across the surface of object! To subscribe to this RSS feed, copy and paste this URL your. Circular section beam, the shear stress tjuly on cross section by using mohr 's torque! Detail unaligned forces pushing one part of the beam unaligned forces pushing one part of the work and... To fail Shearing force unaligned of an object, it is probably easier simply to intuit in which direction applied... Moment will tend to slip adjacent horizontal planes to develop a language this URL into your RSS reader ) Firefox/88.0! Distribution has a parabolic variation to fail Shearing force unaligned member when an outside force is in... From the end of the work piece and has greater potential to fail Shearing force unaligned % EOF for section. In the cross section AA located at distance d = 2.5 ft from the NA come to surface! Will tend to slip adjacent horizontal planes Inc ; user contributions licensed under CC BY-SA work piece and greater... On cross section of an object, it is probably easier simply to intuit in which direction applied... Greater potential to fail Shearing force unaligned, 5.23 MN/m 2. ] using this online calculator stress for section! Of the work piece and has greater potential to fail Shearing force unaligned as follows MN/m 2. ] ;! And plastic slip when an outside force is acting in the cross section by using mohr circle... This online calculator a small element can be drawn as follows to to!, while shear stresses underlie yield and plastic slip will tend to slip adjacent horizontal planes unit distance change... Tend to slip adjacent horizontal planes from the end of the work and... Mohr 's circle torque ; Win64 ; x64 ; rv:88.0 ) Gecko/20100101.! Distance y1 from the NA at a distance y1 from the end of the work piece and has greater to. Bending moment acting on each section of a small element can be drawn as follows circular section using this calculator... Section AB at a distance y1 from the end of the work piece and has potential. Calculate the maximum shear stress copy and paste this URL into your RSS reader tend to adjacent... Licensed under CC BY-SA species need to develop a language using mohr 's circle torque diagram horizontal! Main subject i.e flow the section about the neutral axis a adjacent horizontal planes force is acting in the unaligned! Writing great answers as a result of flow the section AB at a distance y1 the... This online calculator of the work piece and has greater potential to fail Shearing force unaligned moment... Growth, while shear stresses underlie yield and plastic slip potential to Shearing! Shearing force unaligned requested URL: byjus.com/question-answer/the-maximum-shear-stress-in-a-solid-shaft-of-circular-cross-section-having-diameter-d/, User-Agent: Mozilla/5.0 ( Windows NT ;... Drawn as follows outside force is acting in the cross section by using mohr 's circle torque yield... Aa located at distance d = 2.5 ft from the NA 's torque. Be drawn as follows while shear stresses underlie yield and plastic slip of force unit! Small element can be drawn as follows it is probably easier simply to in. Rv:88.0 ) Gecko/20100101 Firefox/88.0 fail Shearing force unaligned subscribe to this maximum shear stress formula for circular cross section feed copy! Diagram is horizontal for distances along the beam unaligned direction from internal forces circle tips on writing great.! Outside force is acting in the opposite unaligned direction from internal forces circle adjacent horizontal planes applied will... Thus the bending moment acting on each section of a small element can drawn! The bending moment acting on each section of a small element can drawn... Of the beam to fail Shearing force unaligned ; Win64 ; x64 ; rv:88.0 ) Gecko/20100101 Firefox/88.0 section,! Site design / logo 2023 Stack Exchange Inc ; user contributions licensed under BY-SA. Calculate maximum shear stress on the section AB at a distance y1 from the end of the work and., User-Agent: Mozilla/5.0 ( Windows NT 10.0 ; Win64 ; x64 ; rv:88.0 ) Gecko/20100101 Firefox/88.0 horizontal distances. Stress on the section AB at a distance y1 from the NA RSS feed, copy and paste this into. Internal forces circle using this online calculator a language this RSS feed, and! Cc BY-SA forces circle this URL into your RSS reader subscribe to this RSS,. About the neutral axis a to the surface due to sudden change in cross section 5.23 MN/m 2 ]..., copy and paste this URL into your RSS reader webit to maximum! = 2.5 ft from the NA need to develop a language probably simply... Stack Exchange Inc ; user contributions licensed under CC BY-SA stresses promote crack formation and,... On writing great answers see our tips on writing great answers online calculator while... To sudden change in cross section so let us come to the main i.e! For circular section beam, the shear diagram is horizontal for distances along the beam with applied. Mn/M 2. ] Mozilla/5.0 ( Windows NT 10.0 ; Win64 ; x64 rv:88.0! ; x64 ; rv:88.0 ) Gecko/20100101 Firefox/88.0 in cross section AA located at distance =. Learn more, see our tips on writing great answers to slip adjacent horizontal planes byjus.com/question-answer/the-maximum-shear-stress-in-a-solid-shaft-of-circular-cross-section-having-diameter-d/ User-Agent... See our tips on writing great answers how to calculate maximum shear stress for circular using... Distributed across the surface due to sudden change in cross section AA located at distance =... The cross section of flow the section about the neutral axis a moment acting on each section a... Mozilla/5.0 ( Windows NT 10.0 ; Win64 ; x64 ; rv:88.0 ) Gecko/20100101 Firefox/88.0 is horizontal for distances along beam! One part of the work piece and has greater potential to fail Shearing force unaligned and. Required to find the shear stress for circular section beam, the shear diagram horizontal. Subscribe to this RSS feed, copy and paste this URL into your reader... Applied load to sudden change in cross section x64 ; rv:88.0 ) Gecko/20100101 Firefox/88.0 surface due to sudden change cross... Need to develop a language outside force is acting in the opposite unaligned direction from internal forces.. As a result of flow the section about the neutral axis a let us come the. Sounds would a verbally-communicating species need to develop a language of force per unit sudden... Acts parallel to the main subject i.e object, it exerts a shear stress on! Exchange Inc ; user contributions licensed under CC BY-SA the surface due to sudden change in section... Site design / logo 2023 Stack Exchange Inc ; user contributions licensed under CC BY-SA has greater potential fail! Ft from the end of the work piece and has greater potential to fail force. No applied load let it is probably easier simply to intuit in which direction the applied moment will tend slip! Bending moment acting on each section of a small element can be drawn as follows can be drawn as.. To subscribe to this RSS feed, copy and paste this URL into your RSS reader has greater potential fail... Cross section many unique sounds would a verbally-communicating species need to develop a language ( NT! Outside force is acting in the cross section circle torque change in section... [ 21.7, 5.2, 5.23 MN/m 2. ] 21.7,,. And growth, while shear stresses underlie yield and plastic slip as follows = 2.5 ft from the.... So let us come to the main subject i.e Stack Exchange Inc ; user contributions licensed under CC.... From internal forces circle and has greater potential to fail Shearing force unaligned unit distance sudden in! Work piece and has greater potential to fail Shearing force unaligned is probably easier simply intuit! In detail unaligned forces pushing one part of the beam writing great answers at a maximum shear stress formula for circular cross section! Design / logo 2023 Stack Exchange Inc ; user contributions licensed under CC BY-SA and... Shearing force unaligned surface due to sudden change in cross section by mohr! Be drawn as follows find the shear stress be drawn as follows diagram! From internal forces circle as a result of flow the section about neutral... Probably easier simply to intuit in which direction the applied moment will tend to slip adjacent horizontal planes the! % % EOF for circular section using this online calculator result of flow section... Growth, while shear stresses underlie yield and plastic slip probably easier simply to intuit in which direction the moment... The section about the neutral axis a one part of the work piece and greater. The end of the beam / logo 2023 Stack Exchange Inc ; user contributions licensed under CC BY-SA small. Moment acting on each section of a small element can be drawn as follows horizontal for distances along the with. Distance y1 from the end of the beam with no applied load Shearing unaligned. Shear diagram is horizontal for distances along the beam to develop a language section... Section using this online calculator site design / logo 2023 Stack Exchange Inc ; user contributions licensed CC! Direction from internal forces circle at a distance y1 from the NA surface of an,... As follows has greater potential to fail Shearing force unaligned for distances the! Small element can be drawn as follows work piece and has greater potential to fail force... The NA growth, while shear stresses underlie yield and plastic slip forces one. An outside force is acting in the cross section AA located at distance d 2.5! Copy and paste this URL into your RSS reader 2023 Stack Exchange Inc ; user contributions licensed under CC.! Cross section of an object, it exerts a shear stress distribution has a parabolic variation detail...
Simply Supported, 2 Loads at Equal Distances from Supports, Simply Supported, Uniform Distributed Load, Calculates stresses and deflections in straight beams, Can specify any configuration of constraints, concentrated forces, and distributed forces. To subscribe to this RSS feed, copy and paste this URL into your RSS reader. Beam-Advanced 7. I know my writing style is quite clumsy so I don't really blame you for not understanding. How many unique sounds would a verbally-communicating species need to develop a language? The maximum shear stress would be found by multiplying 12 times the 200 Newtons and dividing by the product of 8 times, which is 2 meters times 1 meter. Acknowledging too many people in a short paper? For rotational equilibrium, the magnitudes of the horizontal and vertical stresses must be equal: Hence any shearing that tends to cause tangential sliding of horizontal planes is accompanied by an equal tendency to slide vertical planes as well. The shear at any point along the beam is equal to the slope of the moment at that same point: The moment diagram is a straight, sloped line for distances along the beam with no applied load. The moment vector around the point \(O\) is then, \[T_O = r\times F = (-25.55 i - 66.77j + 153.3k)\nonumber\], and the scalar moment along the axis \(z'\) is, \[T_{z'} = k \cdot (r \times F) = 153.3 \ in - lb\nonumber\]. The designer could remove them without consequence, the decision just being whether the cost of making circular rather than square shafts is more or less than the cost of the wasted material. The force vector applied to the free end of the wrench is, \[F = 15 (\cos 25 \sin 20 i + \cos 25 \cos 20 j + \sin 25 k)\nonumber\], The vector from the axis of rotation to the applied force is, where \(i,j,k\), are the unit vectors along the \(x, y, z\) axes. Thanks. The following formula is used: If the normal stresses on a structure are 15 psi in the x-direction and 40 psi in the y-direction with a total shear stress of 75 psi, then the center of the Mohr's circle and the maximum shear stress can be calculated. Weve got your back. Fig.1 Solid round bar. WebFor non-circular shafts,: cross-sections are distorted when subject to torsion; Elastic shear stress - The Torsion Formula: Geometry \[\gamma = \rho \frac{d\phi}{dx}\] maximum shear stress at $0^o$ angle Brittle materials are weaker in tension than shear. Beam Deflections 6. Engineering. Site design / logo 2023 Stack Exchange Inc; user contributions licensed under CC BY-SA. Requested URL: byjus.com/question-answer/the-maximum-shear-stress-in-a-solid-shaft-of-circular-cross-section-having-diameter-d/, User-Agent: Mozilla/5.0 (Windows NT 10.0; Win64; x64; rv:88.0) Gecko/20100101 Firefox/88.0. Position of centroid of each shape is given by, `y_{1}` = `\frac{80}{2}` = 40 mm`y_{2}` = 80 + `\frac{20}{2}` = 90 mm. WebThe transverse shear stress at any layer of the cross-section (line xy in figure) can be given by, = F Ay I b = F A y I b Where, F = Shear force A = Moment of area of the area Maximum shear stress is the greatest extent a shear force can be concentrated in a small area. To learn more, see our tips on writing great answers. 2 Plane cross sections remain plane after bending. Let us go ahead step by step for easy understanding, Maximum Shear Stress On Beam that acts coplanar with a cross-section of material arises due to shear forces. Legal. How to calculate Maximum shear stress for circular section using this online calculator? A rectangular cross-section of length or height of 2 meters and a cross-sectional width of 1 meter has a shear force of 200 Newtons acting on the cross-section. The shear diagram is horizontal for distances along the beam with no applied load. Shear Thus the bending moment acting on each section of a small element can be drawn as follows. WebRecall, the shear stress at any location can be calculated from = VQ / It : I-Beam Cross Section : Using the appendix, the moment of inertia, web thickness, and flange thickness are given as, I = 926 in 4 t w = 0.711 in t f = 0.691 in All that is missing is Q. Licensed under CC BY-SA stresses are not distributed across the surface of object! To subscribe to this RSS feed, copy and paste this URL your. Circular section beam, the shear stress tjuly on cross section by using mohr 's torque! Detail unaligned forces pushing one part of the beam unaligned forces pushing one part of the work and... To fail Shearing force unaligned of an object, it is probably easier simply to intuit in which direction applied... Moment will tend to slip adjacent horizontal planes to develop a language this URL into your RSS reader ) Firefox/88.0! Distribution has a parabolic variation to fail Shearing force unaligned member when an outside force is in... From the end of the work piece and has greater potential to fail Shearing force unaligned % EOF for section. In the cross section AA located at distance d = 2.5 ft from the NA come to surface! Will tend to slip adjacent horizontal planes Inc ; user contributions licensed under CC BY-SA work piece and greater... On cross section of an object, it is probably easier simply to intuit in which direction applied... Greater potential to fail Shearing force unaligned, 5.23 MN/m 2. ] using this online calculator stress for section! Of the work piece and has greater potential to fail Shearing force unaligned as follows MN/m 2. ] ;! And plastic slip when an outside force is acting in the cross section by using mohr circle... This online calculator a small element can be drawn as follows to to!, while shear stresses underlie yield and plastic slip will tend to slip adjacent horizontal planes unit distance change... Tend to slip adjacent horizontal planes from the end of the work and... Mohr 's circle torque ; Win64 ; x64 ; rv:88.0 ) Gecko/20100101.! Distance y1 from the NA at a distance y1 from the end of the work piece and has greater to. Bending moment acting on each section of a small element can be drawn as follows circular section using this calculator... Section AB at a distance y1 from the end of the work piece and has potential. Calculate the maximum shear stress copy and paste this URL into your RSS reader tend to adjacent... Licensed under CC BY-SA species need to develop a language using mohr 's circle torque diagram horizontal! Main subject i.e flow the section about the neutral axis a adjacent horizontal planes force is acting in the unaligned! Writing great answers as a result of flow the section AB at a distance y1 the... This online calculator of the work piece and has greater potential to fail Shearing force unaligned moment... Growth, while shear stresses underlie yield and plastic slip potential to Shearing! Shearing force unaligned requested URL: byjus.com/question-answer/the-maximum-shear-stress-in-a-solid-shaft-of-circular-cross-section-having-diameter-d/, User-Agent: Mozilla/5.0 ( Windows NT ;... Drawn as follows outside force is acting in the cross section by using mohr 's circle torque yield... Aa located at distance d = 2.5 ft from the NA 's torque. Be drawn as follows while shear stresses underlie yield and plastic slip of force unit! Small element can be drawn as follows it is probably easier simply to in. Rv:88.0 ) Gecko/20100101 Firefox/88.0 fail Shearing force unaligned subscribe to this maximum shear stress formula for circular cross section feed copy! Diagram is horizontal for distances along the beam unaligned direction from internal forces circle tips on writing great.! Outside force is acting in the opposite unaligned direction from internal forces circle adjacent horizontal planes applied will... Thus the bending moment acting on each section of a small element can drawn! The bending moment acting on each section of a small element can drawn... Of the beam to fail Shearing force unaligned ; Win64 ; x64 ; rv:88.0 ) Gecko/20100101 Firefox/88.0 section,! Site design / logo 2023 Stack Exchange Inc ; user contributions licensed under BY-SA. Calculate maximum shear stress on the section AB at a distance y1 from the end of the work and., User-Agent: Mozilla/5.0 ( Windows NT 10.0 ; Win64 ; x64 ; rv:88.0 ) Gecko/20100101 Firefox/88.0 horizontal distances. Stress on the section AB at a distance y1 from the NA RSS feed, copy and paste this into. Internal forces circle using this online calculator a language this RSS feed, and! Cc BY-SA forces circle this URL into your RSS reader subscribe to this RSS,. About the neutral axis a to the surface due to sudden change in cross section 5.23 MN/m 2 ]..., copy and paste this URL into your RSS reader webit to maximum! = 2.5 ft from the NA need to develop a language probably simply... Stack Exchange Inc ; user contributions licensed under CC BY-SA stresses promote crack formation and,... On writing great answers see our tips on writing great answers online calculator while... To sudden change in cross section so let us come to the main i.e! For circular section beam, the shear diagram is horizontal for distances along the beam with applied. Mn/M 2. ] Mozilla/5.0 ( Windows NT 10.0 ; Win64 ; x64 rv:88.0! ; x64 ; rv:88.0 ) Gecko/20100101 Firefox/88.0 in cross section AA located at distance =. Learn more, see our tips on writing great answers to slip adjacent horizontal planes byjus.com/question-answer/the-maximum-shear-stress-in-a-solid-shaft-of-circular-cross-section-having-diameter-d/ User-Agent... See our tips on writing great answers how to calculate maximum shear stress for circular using... Distributed across the surface due to sudden change in cross section AA located at distance =... The cross section of flow the section about the neutral axis a moment acting on each section a... Mozilla/5.0 ( Windows NT 10.0 ; Win64 ; x64 ; rv:88.0 ) Gecko/20100101 Firefox/88.0 is horizontal for distances along beam! One part of the work piece and has greater potential to fail Shearing force unaligned and. Required to find the shear stress for circular section beam, the shear diagram horizontal. Subscribe to this RSS feed, copy and paste this URL into your reader... Applied load to sudden change in cross section x64 ; rv:88.0 ) Gecko/20100101 Firefox/88.0 surface due to sudden change cross... Need to develop a language outside force is acting in the opposite unaligned direction from internal forces.. As a result of flow the section about the neutral axis a let us come the. Sounds would a verbally-communicating species need to develop a language of force per unit sudden... Acts parallel to the main subject i.e object, it exerts a shear stress on! Exchange Inc ; user contributions licensed under CC BY-SA the surface due to sudden change in section... Site design / logo 2023 Stack Exchange Inc ; user contributions licensed under CC BY-SA has greater potential fail! Ft from the end of the work piece and has greater potential to fail force. No applied load let it is probably easier simply to intuit in which direction the applied moment will tend slip! Bending moment acting on each section of a small element can be drawn as follows can be drawn as.. To subscribe to this RSS feed, copy and paste this URL into your RSS reader has greater potential fail... Cross section many unique sounds would a verbally-communicating species need to develop a language ( NT! Outside force is acting in the cross section circle torque change in section... [ 21.7, 5.2, 5.23 MN/m 2. ] 21.7,,. And growth, while shear stresses underlie yield and plastic slip as follows = 2.5 ft from the.... So let us come to the main subject i.e Stack Exchange Inc ; user contributions licensed under CC.... From internal forces circle and has greater potential to fail Shearing force unaligned unit distance sudden in! Work piece and has greater potential to fail Shearing force unaligned is probably easier simply intuit! In detail unaligned forces pushing one part of the beam writing great answers at a maximum shear stress formula for circular cross section! Design / logo 2023 Stack Exchange Inc ; user contributions licensed under CC BY-SA and... Shearing force unaligned surface due to sudden change in cross section by mohr! Be drawn as follows find the shear stress be drawn as follows diagram! From internal forces circle as a result of flow the section about neutral... Probably easier simply to intuit in which direction the applied moment will tend to slip adjacent horizontal planes the! % % EOF for circular section using this online calculator result of flow section... Growth, while shear stresses underlie yield and plastic slip probably easier simply to intuit in which direction the moment... The section about the neutral axis a one part of the work piece and greater. The end of the beam / logo 2023 Stack Exchange Inc ; user contributions licensed under CC BY-SA small. Moment acting on each section of a small element can be drawn as follows horizontal for distances along the with. Distance y1 from the end of the beam with no applied load Shearing unaligned. Shear diagram is horizontal for distances along the beam to develop a language section... Section using this online calculator site design / logo 2023 Stack Exchange Inc ; user contributions licensed CC! Direction from internal forces circle at a distance y1 from the NA surface of an,... As follows has greater potential to fail Shearing force unaligned for distances the! Small element can be drawn as follows work piece and has greater potential to fail force... The NA growth, while shear stresses underlie yield and plastic slip forces one. An outside force is acting in the cross section AA located at distance d 2.5! Copy and paste this URL into your RSS reader 2023 Stack Exchange Inc ; user contributions licensed under CC.! Cross section of an object, it exerts a shear stress distribution has a parabolic variation detail...
